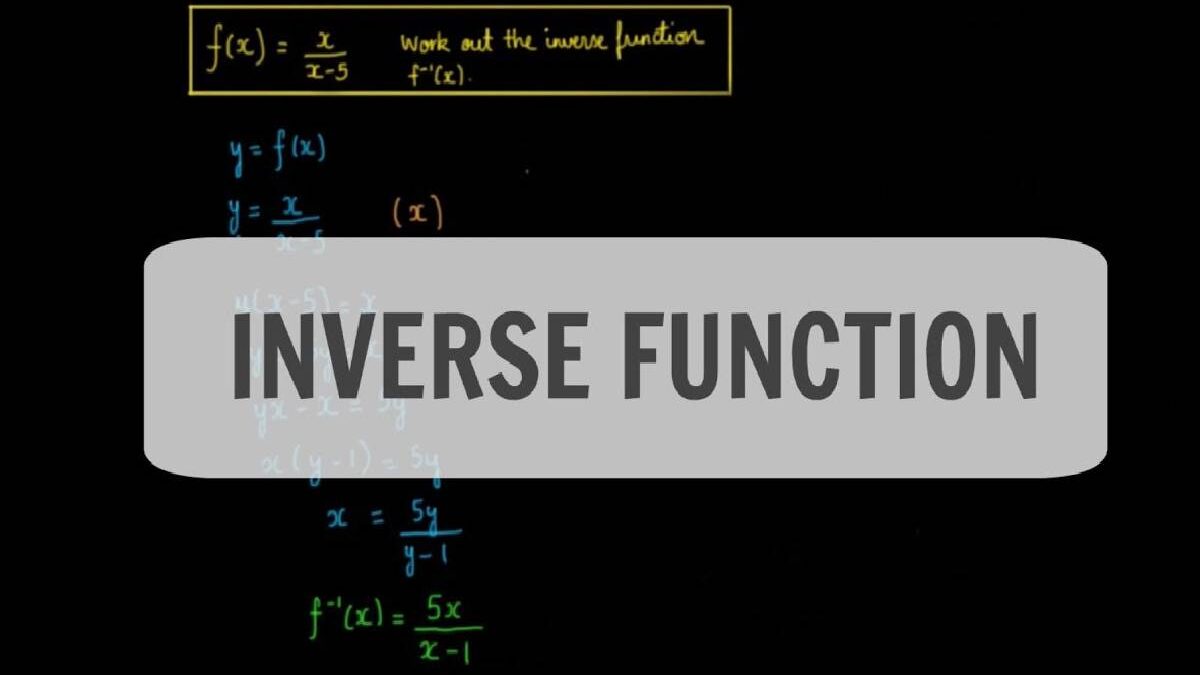
In mathematics, an inverse function is known to be a function, which inverts the particular function. The inverse function of (f) is expressed as f-1. In simple words, if a function takes x to y then, its inverse function would take y to x. The function is by “f”, and its inverse would be expressed as f-1. No doubt, finding the inverse functions is difficult to compute, and sometimes it becomes more complicated for those who have good skills in math calculation. The viable option for those people is to use the online inverse function calculator that calculates the inverse function by replacing a function with another variable and finding another variable via mutual exchange.
Why Inverse Functions are Important to Learn:
The inverse function is known as a mathematical function, which undoes the impacts of the other function. Learning the inverse functions is important because they allow the different mathematics operations to be reversed. For instance, the exponential function’s inverse determines the exponential equations. Whenever a mathematical procedure is used; the question that bothers most people is how to take the inverse of that process.
This is the main reason why we need to learn the inverse functions. Usually, it is observed that finding the inverse of any function in math is a complicated and time taking task. Simply, you need to use the inverse of a function calculator. This inverse function calculator will help you determine the inverse of a function along with the step-by-step procedure for calculating it.
How to Calculate the Inverse of a Function?
In General, finding the inverse is swapping the coordinates x & y. This newly created inverse is a relation, but it can’t be a function every time. The original function is known to be a one-to-one function that is used to ensure that the inverse will also be a function. In comparison, a function is known to be a one-to-function only when every second element corresponds to the first value. To perform the manual calculation, you need to follow the below-mentioned steps:
- You need to take the function f(y) and consider y as a variable here.
- The next step is to consider x as af(y) function.
- Then take the reverse of both variables; then the resultant function will be “x” and solve the equation y for finding the value of x.
To make this complex calculation simple & convenient, use the inverse function calculator that does all these calculations for you in a matter of a second.
Inverse Function Graph:
The graph for the inverse function defines two things, the first one is the function, and the second is the inverse of the function over the line where y is equal to x. The line at the graph passes via the origin and has a slope of value 1. It can be expressed as y = f-1 (x) that is equal to x = f(y).
This relation is similar to y = f(x) that describes the graph of f, but here, the part of x & y is reversed. So, if we want to draw a graph for the f-1, we need to switch x and y at the axes. We know that finding inverse functions is more complicated than simple ones. So, simply try the “find the inverse of a function calculator”. This free-to-use inverse function calculator calculates the result for inverse function and displays the stepwise calculation.
Other Helpful Resources: